Straight Line Mechanisms Theory of Machines
There are a few uncommon mechanisms that may meet common desires of mechanical engineering troubles. these gear assist with a number purposes like generating immediately traces, moving torque between non-coaxial shafts, self centering guidance and mechanical punched card readers.
some mechanisms have special motion characteristics exclusive from the ones of established mechanisms. these mechanisms are used for unique purposes and few precise categories of movement. those mechanisms are unusual enough to be referred to as as special Mechanisms. a few not unusual desires of mechanical engineering exercise are:
- Generation of a straight line motion by linkage mechanism.
- Reproduction of a path traced by one point at another tracing point with a change in scale.
- Transfer of torque and motion between non-coaxial shafts with changing relative alignment.
- Automotive steering mechanisms and suspension mechanisms.
- Indexing: Intermittent timed motion.
Straight Line Mechanisms
Generation of straight line motion using linkage mechanisms has always been a common requirement in machine design Practice. Although exact straight line cannot be generated using simple mechanisms though some simple mechanisms are designed such that they can produce approximate straight lines for short range of motion. These approximate straight line mechanisms has important applications in machine design. These mechanisms were used extensively in classical machines such as steam engines. Perfect straight lines can also be generated using linkage mechanisms but those are relatively complex mechanisms.
There are two classes of straight line mechanisms:
- Approximate Straight Line Mechanisms
- Exact Straight Line Mechanisms
The straight line mechanisms were mostly developed in industrial revolution days when many machines required straight line paths in their operations, whether it was guiding the piston of engines or for operating valves. Straight line mechanisms were developed by continuous effort in trail and error process with making intelligent variations in linkage mechanisms.
Pantograph

Image source
(OA/OE) = (OA'/OE')
which uses at the top of the train
Peaucellier
It is an Exact Straight line Mechanism

Image Source
First, it must be proven that points O, B, D are collinear. This may be easily seen by observing that the linkage is mirror-symmetric about line OD, so point B must fall on that line.
More formally, triangles BAD and BCD are congruent because side BD is congruent to itself, side BA is congruent to side BC, and side AD is congruent to side CD. Therefore, angles ABD and CBD are equal.
Next, triangles OBA and OBC are congruent, since sides OA and OC are congruent, side OB is congruent to itself, and sides BA and BC are congruent. Therefore, angles OBA and OBC are equal.
Finally, because they form a complete circle, we have
- ∠OBA + ∠ABD + ∠DBC + ∠CBO = 360°
but, due to the congruences, angle OBA = angle OBC and angle DBA = angle DBC, thus
- 2 × ∠OBA + 2 × ∠DBA = 360°
- ∠OBA + ∠DBA = 180°
therefore points O, B, and D are collinear.
Hart's Mechanism

ImageSource
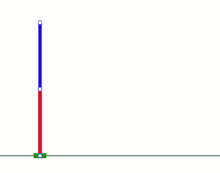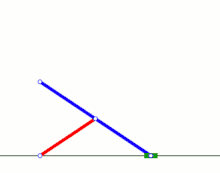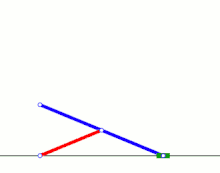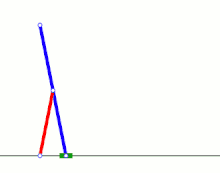
Watt's Straight Line Mechanism

source
Tchebicheff Straight Line Mechanism

 source
sourceScott-Russell Exact Straight Line Mechanism
 source:-wikimedia
source:-wikimedia
I see your main page doesn't rank high in google, but your articles can get into top 10.
ReplyDeleteYou should choose the right longtail keywords before you
write a post. How to find super easy longtail keywords?
Search in google for; Fasrixo's tools